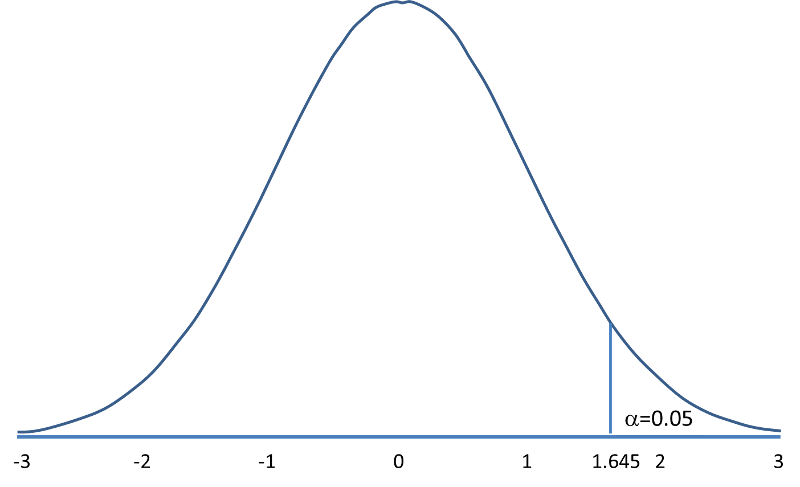
How to Know Which Area in Upper Tail to Use

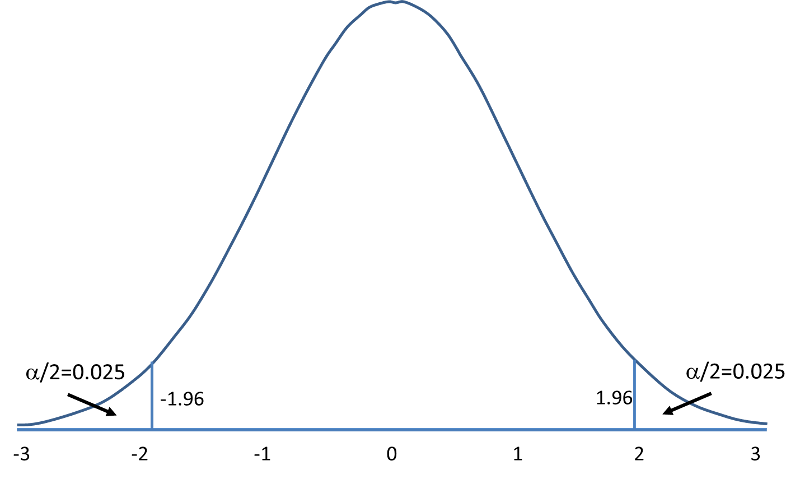
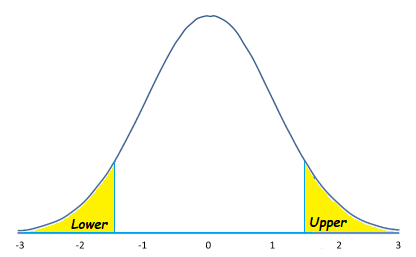
By Da_Rihanna118 24 Apr, 2022 Post a Comment

If Component A has a longer time-to-failure than Component B. In this example however the rejection region must be split between both tails of the distribution0025 in the upper tail and 0025 in the lower tailbecause your hypothesis specifies only a difference not a direction as shown in Figure 1a.

Hypothesis Testing Upper Lower And Two Tailed Tests

If your data follows a normal distribution or if you have a large sample size n 30 that is approximately normally distributed you can use the z -distribution to find your critical values.

. Pval pnorm z lowertailFALSE pval upper tail p value. Two-Tailed Test Lower-Tailed Test Upper-Tailed Test Sign for H0 or or Sign for HA Rejection region In both tails In the left tail In the right tail Ex203 Is the mean different than µ0. 9 2 7 9 0.

The area under the density curve from the left critical value to the left is equal to α2 and the area under the curve from the right critical value to the right is equal to α2 as well. The z-score that corresponds to a value of 6217 in the z-table is 31. For example the area to the left of z 109 is given in the table as 8621.

0 7 2 1 1-0927900721 1 0. We can get this directly with invNorm. Lets say were testing H_0.

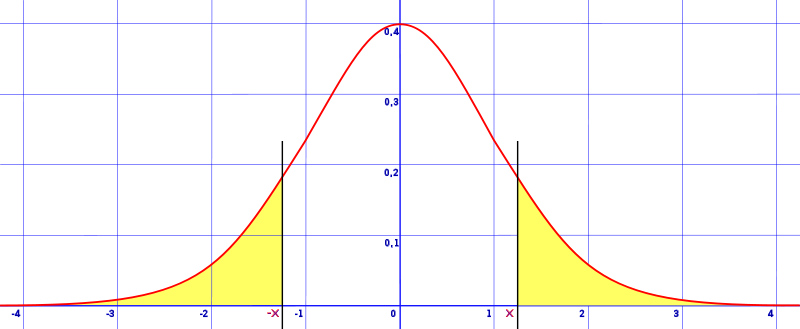
Where μ 0 is a hypothesized upper bound of the true population mean μ. But if the area between the two values is 075 then the total tail area must be 025 square units. Thus total area equals α.

For example your t 160 is between t -values 144 and 194 df 6. Use the Percentile to Z-Score Calculator. Here P-value PrT t.

Next use the positive z-table to find the area to the left of 121. The row near the bottom with Z in the df column gives right-tail greater-than probabilities from the Z -distribution. 0 7 2 1.

So we could use the confidence level to look up the z-value. In such case use Upper Tailed based test. Since the total area under the bell curve is 1 as a decimal value which is equivalent to 100 we subtract the area from the table from 1.

Thus if we know the area to the right is 3783 then the area to the left is 1 3783 6217. P Xltxstar is equal to the area to the left of xstar so we are looking for the cutoff point for a left tail of area 09332 under the normal curve with mean 10 and standard deviation 25. µ µ0 Is the mean lower than µ0.

Let us define the test statistic z in terms of the sample mean the sample size and the population standard deviation σ. The value for alpha can be thought of as the chance of rejecting H_0 the null hypothesis when in fact H_0 is actually true. Look up the critical value that corresponds with the alpha value.

Heres how to use the t -table to find t -values critical. In order to calculate the appropriate area in the upper right tail we must first convert our data to the standard normal distribution. That gives us an area of8869 2266 6603.

Finally subtract the smaller area 2266 from the larger area 8869. Instead of using the critical value we apply the pnorm function to compute the upper tail p-value of the test statistic. The z-score for x 60 is.

When you perform a one-tailed test the entire significance level percentage goes into the extreme end of one tail of the distribution. Thus the area to the left of x 1 must be 0125 square units and the area to the right of x 2 must be 0125 square units. So the right tail probability for your t is between 010 column heading for t 144 and 005 column heading for t 194.

For a two-tailed interval divide your alpha by two to get the alpha value for the upper and lower tails. The area under the density curve from the critical value to the right is equal to α. X µ then rejection region lies on upper tail of the T-Distribution upper-tailed.

First we use the negative z-table to find the area to the left of -75. Notice how lining the two normal curves up as shown illustrates how the two areas are the same. Thus the area to the right of z 109 is 1 - 8621.

In the examples below I use an alpha of 5. As we know that the area of the curve excluding the tails is 095 or 95 that means the confidence level of the statement is 95. µ µ0 HA.

9 2 7 9 0. The null hypothesis of the upper tail test of the population mean can be expressed as follows. One-tailed hypothesis tests are also known as directional and one-sided tests because you can test for effects in only one direction.

We can also use the area in one of the curves which is 0025 to look up the z-value. Then the null hypothesis of the upper tail test is to be rejected if z z α where z α is the. PX 60 PZ 125.

This means that 60 is 125 standard deviations above the mean. As it turns out to be greater than the 05 significance level we do not reject the null hypothesis that p 012. µ µ0 HA.

µ µ0 Is the mean lower or still the same than µ0. Use a z-table to reverse-lookup the z-value that gives the desired upper-tail area that area is alpha for a one-tailed test and alpha2 for a two-tailed test. As in the last example you decide to use a 5 percent probability level for the test.

The z table shows the area to the left of various z-scores. Both tests have a region of rejection then of 5 percent or 005. To find the area to the right you need to subtract the value in the z z z -table from 1 1 1.

49 rows T-Statistics Reference Table - Upper Tailed Test - alpha Area under the curve for. This means that we have P X x 1 0125 and P X x 2 0125. If the mean of the sample of interest is greater than the comparison mean.

But in an upper-tail test youre interested in the area to the right of the z z z -value not the area to the left. The R statements to get these values are qchisq 0125 11 and qchisq 0125 11 lowertailFALSE. In a z z z -table you find.

Hypothesis Testing Upper Lower And Two Tailed Tests